12肥料经济学--肥料经济学(ⅰ)--最大利润与最高产量 18页

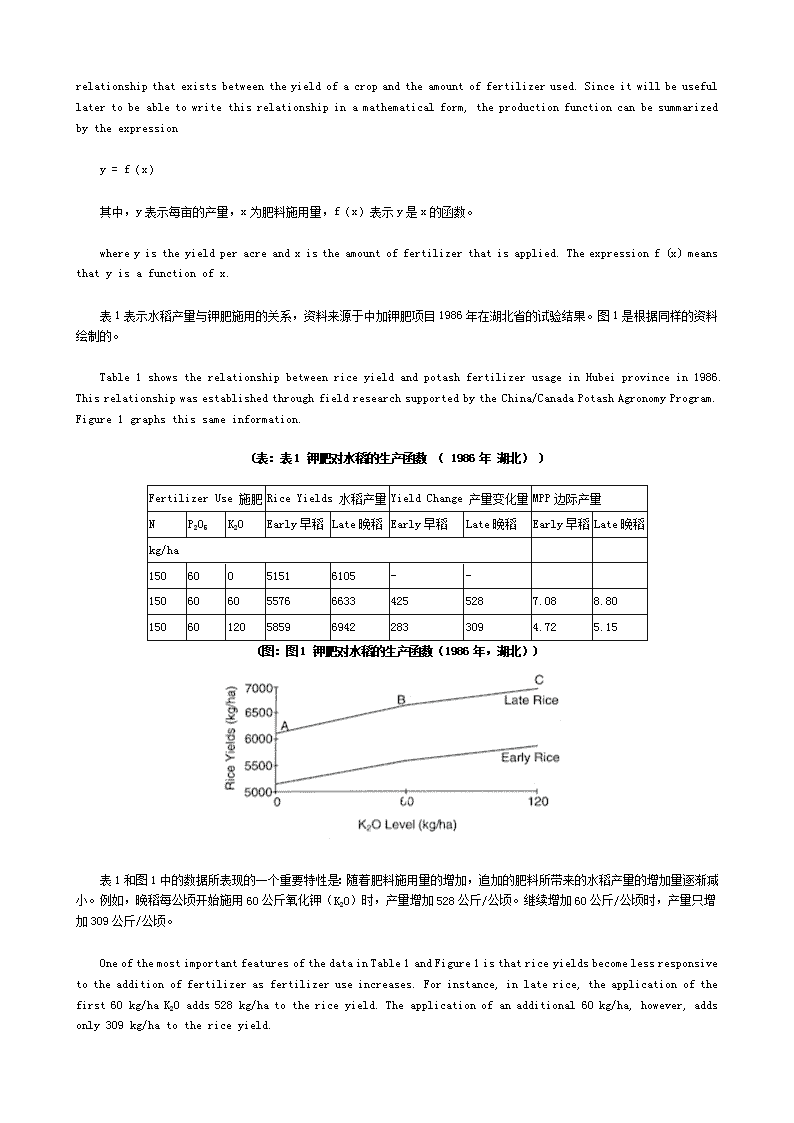
肥料经济学（Ⅰ）--最大利润与最高产量MurrayFulton教授加拿大萨斯卡彻温大学农经系、合作研究中心　　引言Introduction　　肥料对中国的农业和经济具有极为重要的意义。过去40年来中国农业增长的主要原因之一是肥料使用量的增加。目前中国的化肥年使用量约9千万吨（以产品重量计），成为世界上化肥消费的大国之一。同时，中国也是世界上进口化肥最多的国家，进口量约为1500万吨。　　FertilizerisextremelyimportanttoChineseagricultureandtotheChineseeconomy.Oneofthemajorreasonsfortheincreaseinagriculturalproductionoverthelast40yearsistheincreaseinuseoffertilizer.CurrentlyChinaconsumesapproximately90millionmetrictons(mmt)ofchemicalfertilizer(measuredinproductionweight).ThismakesChinaoneofthelargestconsumersoffertilizerintheworld.Chinaisalsothelargestimporteroffertilizer,importingroughly15mmtofchemicalfertilizer.　　除了为作物生产提供养分外，化肥生产和运销在中国经济中也具有重要意义。例如，中国现有近1800个化肥厂，从业人员达220万，政府每年花费数十亿元用于肥料的生产和分销，化肥进口也是中国外汇硬通货支出的重要项目之一。　　Inadditiontoprovidingthenutrientsrequiredforcropproduction,fertilizerproductionandtransportationplayanimportantroleintheChineseeconomy.Thenearly1,800fertilizerplantsinChina,forinstance,employ2.2millionworkers.Governmentinvestmentintofertilizerproductionanddistributioninvolvesexpendituresofbillionsofyuanperyear,whiletheimportationoffertilizerisoneofthemajorusesofhardforeigncurrency.　　肥料生产经济学TheEconomicsofFertilizerProduction　　经济学的主要特性之一是着重研究：为达到某一目标，如何最有效地分配和利用资源，不管是资金、劳动力或肥料。经济学的一个特点是可以用同样的概念和方法针对多种不同的问题或目标进行分析。　　Akeyfeatureofeconomicsisitsfocusonhowbesttoallocateresources-whetherthisbemoney,orlabour,orfertilizer-inordertoachievesomegoal.Oneofthelessonsofeconomicsisthatalargenumberofproblems-ie.,manydifferentgoals-canbeanalysedwiththesamesetoftoolsandconcepts.　　具体地说，经济学可对肥料使用中两个完全不同的问题做出解答。其一是应该使用多少肥料以获得最大量的物质指标，如单产量或总产量；其二是应该使用多少肥料以获得最大量的经济指标，如农户的利润，或者一个省或一个国家的纯经济效益。　　Inparticular,economicscanprovideananswertotwoverydifferentquestionsthatareoftenraisedwithrespecttofertilizer.Thefirstconcernshowmuchfertilizershouldbeusedtomaximizeaphysicalvariablesuchasyieldortotalproduction.Thesecondconcernshowmuchfertilizershouldbeusedtomaximizeaneconomicvariablesuchastheprofitsofthefarmerortheeconomicnetbenefitsofaprovinceora\ncountry.　　要对这两个问题做出回答，通常的方法是从边际分析入手。就肥料的使用而言，边际分析的重要一步是要了解产量曲线或生产函数。如下面将要显示的，产量曲线的形状与边际收益递减的概念有着密切的关系。　　Thecommonapproachtoansweringbothofthesequestionsinvolvestheapplicationofwhatisknownasmarginalanalysis.Inthecaseoffertilizer,animportantpartofmarginalanalysisinvolvesanunderstandingoftheshapeoftheyieldresponsecurve,orproductionfunction.Aswillbeshown,theshapeoftheyieldresponsecurveiscloselyrelatedtotheideaofdiminishingmarginalreturns.　　鉴于生产函数的重要性，这里首先对它进行分析。在对产量和肥料之间的物质关系了解之后，再对最高产量和最大利润进行阐述。同时，还将对产量优化和利润优化的不同解决方法进行讨论。　　Becauseofitsimportance,theproductionfunctionisexaminedfirst.Oncethenatureofthephysicalrelationshipbetweenfertilizerandyieldisunderstood,theanalysisshiftstoanexaminationofthequestionofmaximizingyield,followedbyanexaminationofprofitmaximization.Anexplanationofthedifferencebetweenthesolutionstothesetwoproblemsisalsodiscussed.　　在展开前面所提及的概念时，会用到中国农业的一些例子，这些资料大部分来源于加拿大钾磷研究所的研究结果，主要涉及钾肥的施用。　　Indevelopingtheconceptsoutlinedabove,examplesfromChineseagriculturewillbeused.ManyoftheexampleswillbetakenfromworkdonebythePotashandPhosphateInstituteofCanada(PPIC)andwillinvolvetheuseofpotash.　　这个讲座所介绍的概念带有理想化的色彩，也就是说，我们假设肥料的使用是没有限制的。下一个讲座，即“肥料经济学（Ⅱ）”，将讨论在存在限制因素的情况下怎样进行分析。　　Theconceptspresentedinthislecturewillconsideranidealworldinwhichtherearenoconstraintstotheuseoffertilizer.Thenextlecture,EconomicsofFertilizerⅡ,willdiscusshowtheanalysisneedstobechangedinordertoexaminethepresenceofconstraints.　　在讨论合理施肥的经济问题时，我要强调这些讲座主要以概念论述为主。也就是说，我们将着重考虑肥料使用中的问题而不涉及细节。因此对于诸如价格或产量的不稳定性、施用时间、施肥位置和深度以及环境因素如有效土壤湿度的影响等等将不予讨论。这些问题都很重要，很多专著已作了研究，在此过多考虑它会分散对本讲座经济学思维框架主题的讨论。　　Indiscussingtheeconomicsoffertilization,Ishouldstressthatthelectureswillbelargelyconceptualinnature.BythisImeantheywillfocusonawayofthinkingaboutproblemsregardingfertilizeruse,ratherthanprovidingagreatnumberofspecifics.Asaresult,Iwillnotbediscussingfactorssuchastheimpactofpriceoryielduncertainty,thetiming,depthandplacementoffertilizerapplication,northeeffectofenvironmentalfactorssuchasmoistureavailability.Whiletheseareimportanttopicsaboutwhichagreatdealhasbeenwritten,considerationofthemwoulddetractfrommymainpurposeofprovidingaframeworkforthinkingaboutproblemsinaneconomicfashion.　　生产函数TheProductionFunction　　生产函数也称产量反应曲线，在这里表示作物产量与肥料施用量之间的内在关系。它在以下讨论中非常有用，故应以数学形式表示，其表达式如下：y=f（x）　　Theproductionfunctionisalsoknownastheyieldresponsecurve.Theproductionfunctionshowsthe\nrelationshipthatexistsbetweentheyieldofacropandtheamountoffertilizerused.Sinceitwillbeusefullatertobeabletowritethisrelationshipinamathematicalform,theproductionfunctioncanbesummarizedbytheexpression　　y=f（x）　　其中，y表示每亩的产量，x为肥料施用量，f（x）表示y是x的函数。　　whereyistheyieldperacreandxistheamountoffertilizerthatisapplied.Theexpressionf(x)meansthatyisafunctionofx.　　表1表示水稻产量与钾肥施用的关系，资料来源于中加钾肥项目1986年在湖北省的试验结果。图1是根据同样的资料绘制的。　　Table1showstherelationshipbetweenriceyieldandpotashfertilizerusageinHubeiprovincein1986.ThisrelationshipwasestablishedthroughfieldresearchsupportedbytheChina/CanadaPotashAgronomyProgram.Figure1graphsthissameinformation.(表：表1钾肥对水稻的生产函数（1986年湖北）)FertilizerUse施肥RiceYields水稻产量YieldChange产量变化量MPP边际产量NP2O5K2OEarly早稻Late晚稻Early早稻Late晚稻Early早稻Late晚稻kg/ha15060051516105--1506060557666334255287.088.8015060120585969422833094.725.15(图：图1钾肥对水稻的生产函数（1986年，湖北）)　　表1和图1中的数据所表现的一个重要特性是：随着肥料施用量的增加，追加的肥料所带来的水稻产量的增加量逐渐减小。例如，晚稻每公顷开始施用60公斤氧化钾（K2O）时，产量增加528公斤/公顷。继续增加60公斤/公顷时，产量只增加309公斤/公顷。　　OneofthemostimportantfeaturesofthedatainTable1andFigure1isthatriceyieldsbecomelessresponsivetotheadditionoffertilizerasfertilizeruseincreases.Forinstance,inlaterice,theapplicationofthefirst60kg/haK2Oadds528kg/hatothericeyield.Theapplicationofanadditional60kg/ha,however,addsonly309kg/hatotherice\nyield.　　产量的增加量随着肥料施用的增加而逐渐减少的趋势称为边际报酬递减。使用边际物质产量（MPP）的定义将使这一概念的表述更加简明:　　Thetendencyfortheyieldresponsetobecomelessasfertilizeruseincreasesisknownasdiminishingmarginalreturns.Theconceptofdiminishingmarginalreturnscanbemademoresuccinctbydefiningthemarginalphysicalproduct(MPP)　　边际物质产量(MPP)=作物产量的变化量/肥料用量的变化量　　MarginalPhysicalProduct(MPP)=ChangeinCropYield/ChangeinFertilizerUse　　表1所示为湖北省早、晚稻钾肥施用的边际产量。计算得到一定肥料用量水平的边际产量。公式中的肥料用量变化量是指一定肥料用量水平与上一用量水平之差。作物产量变化量是指这两个肥料水平对应的两个产量的差值。　　Table1showsthecalculationoftheMPPforpotashonearlyandlatericeinHubei.TheMPPiscalculatedforagivenleveloffertilizeruse.ThechangeinfertilizerusedinthecalculationofMPPisthedifferencebetweenthegivenleveloffertilizeruseandthenextsmallerleveloffertilizeruse.Thechangeinyieldiscalculatedasthedifferencebetweentheyieldsatthesetwofertilizerusagelevels.　　例如，用309除以60而得的边际产量为5.15。其中，309是6942与6633之差，它们是钾肥用量分别为120和60公斤/公顷时的相应产量，60是120与60之差。　　Forexample,theMPPof5.15iscalculatedbydividing309by60.Thevalueof309isthedifferencebetween6942and6633,theyieldsatpotashapplicationlevelsof120and60kg/ha,respectively.Thevalueof60isthedifferencebetween120and60.　　应该强调的是，边际产量的计算并非是用一定的肥料用量水平下的产量与不用肥料的情况下的产量之差除以总的肥料用量。以表1为例来说，用837（即6942-6105）除以120（即120-0）而得出的6.98并不是边际产量。尽管这个数值在计算价值成本比（VCR）时很重要，但在肥料经济学中却并没有实际意义。下面我们会看到，用价值成本比来考察肥料经济会导致极不正确的结论。　　ItshouldbestressedthatthecalculationoftheMPPdoesnotinvolvedividingthedifferencebetweentheyieldatagivenleveloffertilizerandthebaseyieldwhennofertilizerisappliedbythetotalamountoffertilizerused.IntheexampleinTable1,thislatternumberisobtainedbydividing837(6942-6105)by120(120-0)togive6.98.WhilethisnumberplaysanimportantroleinthecalculationoftheValueCostRatio(VCR),itplaysnousefulroleinunderstandingtheeconomicsoffertilizerapplication.Aswillbeshownbelow,theVCRcanproduceextremelymisleadingresultswhenitcomestoconsideringtheeconomicsoffertilization.　　当肥料用量水平差值较大时，通常考虑计算超出两个肥料用量水平范围的边际产量。例如，表1应该解释为，当晚稻的钾肥用量为0~60公斤/公顷时，边际产量为8.80；当钾肥用量为60~120公斤/公顷时，边际产量为5.15。　　Whenthedifferenceintheleveloffertilizeruseislarge,itisusuallyassumedthattheMPPthatiscalculatedappliesovertherangebetweenthetwolevelsoffertilizeruse.Forexample,Table1shouldbeinterpretedassayingthattheMPPoflatericeis8.80forfertilizerapplicationlevelsbetweenzeroand60kg/ha.Forfertilizerapplicationlevelsbetween60and120kg/ha,theMPPis\n5.15.　　边际产量的重要性在于它表示了肥料的追加用量所带来的产量的增量，如表1中所表现的逐渐下降的边际产量说明，肥料用量的增加所带来的增产幅度越来越小。简言之，边际产量表示，每增加一个单位的肥料用量在产量增加中的作用如何。　　TheimportanceoftheMPPisthatitshowswhattheincrementalyieldisfromanadditionalapplicationoffertilizer.Forinstance,thefallingMPPpresentedinTable1indicatesthattheincreaseinyieldthatresultsfromanincreaseinfertilizerusageisbecomingsmallerandsmaller.Inshort,theMPPprovidesanindicationofhoweffectiveadditionalunitsoffertilizerareinraisingyield.　　从图形上看，生产函数曲线的斜率即为边际产量。图1中，线段AB的斜率是钾肥用量为0~60公斤/公顷时的边际产量，线段BC的斜率是钾肥用量范围为60~120公斤/公顷时的边际产量。图2绘出早、晚稻的钾肥边际产量与肥料水平的关系，这些曲线斜率的下降表明了边际报酬递减。　　Graphically,theMPPisgivenbytheslopeoftheproductionfunction.InFigure1,theslopeofthelineABgivestheMPPofpotashovertherangeofpotashusezeroto60kg/ha,whiletheslopeofthelineBCgivestheMPPofpotashovertherangeofpotashuse60to120kg/ha.Figure2graphstheMPPofpotashagainsttheleveloffertilizerforbothearlyandlaterice.Thedownwardslopesofthesecurvesindicatediminishingmarginalreturns.(图：图2钾肥对水稻的边际产量（1986年，湖北）)　　图3表示的是钾肥的一般生产函数。用数学的术语来表示，边际产量是生产函数的一阶导数，即：　　Figure3presentsamoregeneralproductionfunctionforpotash.Inmathematicalterms,themarginalphysicalproductisgivenbythefirstderivativeoftheproductionfunction,ie.,\n(图：图3钾肥对水稻的一般生产函数)　　因此，线段AB的斜率为MPP1，是钾肥用量为K1时的边际产量；线段CD的斜率为MPP2，是钾肥用量为K2时的边际产量。钾肥用量为K3时，边际产量为负值，即MPP3＜0。再说明一下，边际产量与肥料用量水平的关系可以用图表示，边际报酬递减表明边际产量曲线应该向下倾斜。　　Thus,theslopeofthelineABisMPP1,themarginalphysicalproductoffertilizerataleveloffertilizeruseK1,whiletheslopeofthelineCDisMPP2,themarginalphysicalproductoffertilizeratusagelevelK2.AtfertilizerusageK3,theMPPbecomesnegative,ie.,MPP3＜0.Onceagain,theMPPcanbegraphedagainsttheleveloffertilizerusage.DiminishingmarginalreturnsindicatethattheMPPcurvewillbedownwardsloping.　　图4可用来表示边际产量与用于计算价值成本比（VCR）的主要成份之间的关系。假定肥料的三个用量水平为K1、K2和K3，相应的产量分别为Y1、Y2和Y3。Y0为不施用肥料时的产量水平。计算价值成本比的第一步是计算下列比值：(Y1-Y0)/K1，(Y2-Y0)/K2，(Y3-Y0)/K3。这些比值分别为斜线Y0a、Y0b和Y0C。从图4中可以看到，这些线段的斜率和切线AB、CD及EF的斜率没有任何的关系。例如，肥料用量为K3时，边际产量为负值，而(Y3-Y0)/K3却是正值。　　Figure4canbeusedtoshowtherelationshipbetweentheMPPandamajorcomponentusedinthecalculationofVCR.Supposethatthreelevelsoffertilizerusehavebeenobserved-K1,K2,andK3.AssociatedwiththeselevelsoffertilizeruseareyieldsY1,Y2,andY3,respectively.YieldlevelY0istheyieldthatresultswhennofertilizerisapplied.ThefirststepincalculatingtheVCRistocalculatethevalueoftheratios:(Y1-Y0)/K1,(Y2-Y0)/K2,and(Y3-Y0)/K3.ThevaluesoftheseratiosaregivenbytheslopesofthelinesY0a,Y0b,andY0c.AscanbeseenfromFigure4,theslopeoftheselinesbearsnorelationatalltotheslopeofthetangentlinesAB,CD,andEF.Forexample,atfertilizerusagelevelK3,theMPPhasbecomenegative.However,theratio(Y3-Y0)/K3ispositive.\n(图：图4计算价值成本比所需要的要素)　　多种肥料的生产函数ProductionFunctionwithMultipleFertilizers　　在研究肥料用量与作物产量之间的关系时，有必要明确有关的重要变量。例如图1和表1的数据表示两个生产函数，一个是早稻，一个是晚稻。另外，这两个生产函数都是在一定的氮磷肥用量水平下，按照某个特定的省（湖北）在某个特定年份（1986）的实验而得出。　　Whenpresentingtherelationshipbetweenfertilizeruseandcropyield,itisimportantthatallthevariablesimportantintherelationshipbeunderstood.Forinstance,Table1andFigure1presentdatafortwoproductionfunctions,oneforEarlyRiceandoneforLateRice.Inaddition,theproductionfunctionsshownarebasedonagivenamountofnitrogenandphosphorusfertilizerandarebasedontrialsinaparticularprovince(Hubei)inaparticularyear(1986).　　生产函数可以通过显示产量和所研究的各种肥料不同用量水平之间的关系，来说明不同肥料之间的相互作用。例如，表2是施用氮和钾与黄麻产量的关系，资料来源于中加钾肥项目1986年在广东省的大田试验。　　Productionfunctionscanaccountfortheinteractionbetweendifferenttypesoffertilizerbyshowingtherelationshipbetweenyieldandthelevelofusageofallthefertilizersunderconsideration.Asanexample,Table2showstherelationshipbetweentheyieldofjuteandtheamountofnitrogenandpotashapplied.ThedataisforGuandongin1986andwasestablishedthroughfieldresearchsupportedbytheChina/CanadaAgronomyProgram.(表：表2黄麻产量作为N和K2O用量的函数（1986年，广东）)K2OLevel施钾量Yield(kg/ha)产量MPPofPotash钾的边际产量MPPofNitrogen氮的边际产量(kg/ha)N=150N=225N=150N=225035853532-0.7755160518221.022.00.31506015691411.423.112.0225613571071.62.613.0　　图5画的是多个生产要素的生产函数关系。为了便于理解，可以把图5看成是6个单生产要素的生产函数：2个为钾在2个不同的氮水平上，4个为氮在4个不同的钾水平上。线段ABCD和A'B'C'D'表示钾的两个生产函数，线段AA'、BB'、CC'和DD'表示氮的四个生产函数。　　Figure5graphsthisrelationshipinamultipleinputproductionfunction.ItisusefultothinkofFigure5intermsofsixsingleinputproductionfunctions:twoforpotashgiventhetwodifferentlevelsofnitrogenapplication;andfourfornitrogengiventhefourdifferentlevelsofpotashapplication.ThelinesABCDand\nA'B'C'D'showthetwoproductionfunctionsforpotash,whilethelinesAA',BB',CC',andDD'showthefourproductionfunctionsfornitrogen.(图：图5氮肥和钾肥对黄麻的生产函数（1986年，广东）)　　六个单生产要素的生产函数可计算六组边际产量，例如，在氮用量为150公斤/公顷时，可计算一组钾的边际产量，在氮用量为225公斤/公顷时，可计算另一组边际产量。表2提供了两组边际产量值。　　ThesixsingleinputproductionfunctionsallowforthecalculationofsixsetsofMPPs.Forinstance,onesetofMPPscanbecalculatedforpotash,giventhattheapplicationofnitrogenis150kg/ha,whileanothersetofMPPscanbecalculatedforpotash,giventhattheapplicationofnitrogenis225kg/ha.Table2presentsthetwosetsofMPPs.　　当氮用量为150公斤/公顷时，黄麻产量对钾的用量在所有的钾用量水平均呈边际报酬递减。而在氮用量为225公斤/公顷时，黄麻产量起初对钾用量出现边际报酬递增。这是因为，边际产量在钾用量为75~150公斤/公顷时比钾用量为0~75公斤/公顷时大。然而，在钾用量为150~225公斤/公顷时，又出现边际报酬递减，在此钾肥施用范围，边际产量减至2.6。　　Foranitrogenapplicationof150kg/ha,juteyieldsexperiencediminishingmarginalreturnstopotashapplicationoveralllevelsofpotashapplication.Foranitrogenapplicationof225kg/ha,however,juteyieldsinitiallyexperienceincreasingmarginalreturnstopotashapplication.ThisfollowsbecausetheMPPforpotashusebetween75and150kg/haisgreaterthantheMPPforpotashusebetweenzeroand75kg/ha.Forpotashapplicationsbetween150kg/haand225kg/ha,however,therearediminishingmarginalreturnstopotashapplication.Inthisrangeoffertilizeruse,theMPPfallsto2.6.　　图5中，当氮的用量为150公斤/公顷时，钾用量的边际报酬递减反映在生产函数ABCD的斜率随着钾肥用量的增加而逐渐变小。当氮用量为225公斤/公顷时，边际报酬的递增表现为生产函数的斜率从A'到B'，再到C'的逐渐增加，从C'到D'斜率的下降表明边际报酬开始递减。　　InFigure5,thediminishingmarginalreturnstopotashapplicationwhennitrogenapplicationis150kg/haarereflectedbytheslopeoftheproductionfunctionABCDbecomingsmallerandsmallerastheamountofpotashappliedincreases.Foranitrogenapplicationlevelof225kg/ha,increasingmarginalreturnsareshownbytheincreaseintheslopeoftheproductionfunctionasonemovesfromA'toB'toC'.ThefallintheslopeasonemovestoD'indicatesthatdiminishingmarginalreturnshavesetin.\n　　表2又表示在钾的各个用量水平，氮肥用量的变化对钾肥边际产量的影响。特别是氮肥用量的增加具有提高钾肥边际产量的作用。例如，当钾肥用量为75~150公斤/公顷时，氮肥用量由150公斤/公顷增加到225公斤/公顷使得钾肥的边际产量由11.4增至23.1。　　Table2alsoshowsthatachangeinthelevelofnitrogenapplicationaffectstheMPPofpotashuseforanygivenlevelofpotashuse.Inparticular,anincreaseintheamountofnitrogenappliedhastheeffectofincreasingtheMPPofpotash.Asanexample,whenpotashuseisbetween75kg/haand150kg/ha,anincreaseinnitrogenapplicationfrom150to225kg/haincreasestheMPPofpotashfrom11.4to23.1.　　没有足够的数据来证明黄麻的产量是否对氮肥施用亦呈边际报酬递减。然而，表2表明，当不施钾时，氮肥的边际产量为负值，也就是说，氮用量由150公斤/公顷增加到225公斤/公顷导致了黄麻产量的降低，而随着钾用量的增加，氮肥的边际产量也增加。　　Insufficientdataisavailabletodeterminewhetherjuteyieldsarealsoexperiencingdiminishingreturnstonitrogenapplication.Table2shows,however,thatwhennopotashisapplied,themarginalphysicalproductofnitrogenisnegative--thatis,anincreaseinnitrogenusefrom150kg/hato225kg/haleadstoadecreaseinjuteyields.Astheamountofpotashappliedincreases,theMPPofnitrogenincreases.　　氮肥的边际产量随着钾肥用量的增加而增加的现象说明，钾肥对氮肥施用的有效性具有积极的作用。如前所述，氮肥对钾肥的有效性同样具有重要作用。这种内在关系的重要性在于，在决定某种肥料的用量时，必须同时考虑到其它肥料的用量。这种相互依存关系在后面还会作进一步的分析。　　TheincreaseintheMPPofnitrogenaspotashapplicationlevelsareincreasedisanindicationofthepositiveimpactthatpotashhasontheeffectivenessofnitrogen.Aswasjustdiscussed,nitrogenalsohasanimportanteffectontheeffectivenessofpotash.Theimportanceofthisinterrelationshipisthatdecisionsabouthowmuchofonefertilizertousecannotbemadeindependentlyofthedecisionregardingtheamountoftheother.Thisinterdependencewillbefurtherexaminedatalaterpoint.　　图6表示一个氮和钾两种肥料的一般生产函数。这个生产函数可用数学形式表示为　　y=f(N，K)　　Figure6presentsageneralproductionfunctionfortwofertilizers,potashandnitrogen.Theproductionfunctioncanbewrittenmathematicallyas　　y=f(N，K)\n(图：图6两种相互作物的肥料的一般生产函数)　　氮肥的边际产量（MPPN）是这个函数在“氮方向”的斜率，因而，在氮肥用量为N*时，MPPN等于线段CD的斜率。钾的边际产量（MPPK）是这个函数在“钾方向”的斜率，即MPPK等于线段AB的斜率。　　TheMPPofnitrogen(MPPN)isgivenbytheslopeofthefunctioninthe"Ndirection."Thus,atN*,MPPNisequaltotheslopeoflineCD.TheMPPofpotash(MPPK)isgivenbytheslopeofthefunctioninthe"Kdirection,"ie.,MPPKisequaltotheslopeoflineAB.　　数学上，这些边际产量是生产函数的偏导数，　　Mathematically,theMPPsaregivenbythepartialderivativesoftheproductionfunction.Thus,　　边际报酬递减是说对N和K的偏导数分别随着N和K的增加而减少。　　DiminishingmarginalreturnsimpliesthatthepartialderivativeswithrespecttoNandKdecreaseasNandK,respectively,increase.　　最高产量OutputMaximization　　如我在引言中提到的，边际报酬递减的概念是理解肥料经济学的关键。报酬递减表明，在一定程度上，肥料单位用量的增加不再对产量产生影响，即产量增量为零。如果产量不再增加，那一定是达到了最高产量。由于产量增加为零也意味着边际产量为零，达到最大产量时也就是肥料的边际产量为零的时候。图7表明，当肥料用量为Kmax时，生产函数的斜率（线段EF的斜率）为零，即边际产量为零，因而，Kmax便为获得最高产量的肥料用量。　　AsImentionedinmyintroduction,theconceptofdiminishingmarginalreturnsplaysakeyroleinunderstandingtheeconomicsoffertilization.Diminishingreturnssuggestthatatsomepointanadditionalunitoffertilizernolongerhasanyimpactonyield--i.e.,theyieldresponseiszero.Ifyieldcannotbeincreased,thenthemaximumyieldmustbeobtained.SinceazeroyieldresponsealsomeanstheMPPiszero,thenthemaximumyieldisattainedwhentheMPPoffertilizeriszero.Figure7showsthatatfertilizerlevelKmaxtheslopeoftheproductionfunction(theslopeofthelineEF)iszeroandtheMPPiszero.FertilizerlevelKmaxisthereforetheleveloffertilizerusagethatmaximizesproduction.\n(图：图7钾肥对水稻的一般生产函数)　　当两种或两种以上的肥料相互作用而影响产量时，寻找取得最高产量的肥料用量的方法也是相似的。在这种情况下，最高产量产生在两种肥料的边际产量都等于零的时候。因为，如果两个边际产量都为零，说明产量对任何一种肥料的施用都不再做出反应。　　Themethodoffindingtheleveloffertilizerusethatmaximizesproductionissimilarwhentwoormorefertilizersinteracttoaffectyield.Inthissituation,maximumyieldoccurswhentheMPPforbothfertilizersareequaltozero.ThisisbecauseifbothMPPsareequaltozero,thenyieldnolongerrespondstotheapplicationofeitherfertilizer.　　图8表示的是产量为氮肥和钾肥施用的函数。当“钾肥方向”的线段斜率（即线段AB）和“氮肥方向”的线段斜率（即线段CD）同时为零时，取得最高产量，其结果是钾的用量为Kmax和氮的用量为Nmax时，产量最高。　　Figure8presentsageneralproductionfunctionthathasyieldasafunctionofnitrogenandpotashuse.Maximumyieldisattainedwhentheslopeofthelineinthe"potashdirection"(i.e.,lineAB)isequaltozeroandwhentheslopeofthelineinthe"nitrogendirection"(i.e.,lineCD)isequaltozero.Asaresult,anapplicationofKmaxofpotashandNmaxofnitrogenwillmaximizeyield.(图：图8两种肥料的生产函数达到最高产量的肥料用量水平)　　最大利润Profit\nMaximization　　经济学家们通常认为，在大多数情况下最高产量并不是一个适当的目标。而更相信投入值和产出值有某种关系。因为投入的生产要素一般来说不是免费的，需要消耗资源，无论这种消耗是金钱、时间或放弃生产其它可供选择的产品，生产要素的价值即为成本。另一方面，产品是对资源的潜在索求，无论是以金钱或以其它产品的形式，产品的价值即为收益。　　Economistsusuallysuggestthatmaximumproductionisnotanappropriateobjectiveinmostsituations.Instead,theybelievethatanobjectivethatvaluesinputsandoutputsinsomemannerispreferred.Sinceinputsaregenerallynotfree,butrequiretheexpenditureofresources,whetheritbeintermsofmoney,time,orthegivingupofotherproductionthatcouldhavebeenproduced,thevalueofinputsisconsideredtobeacost.Outputs,ontheotherhand,representapotentialclaimonresources,whetheritbeintermsofmoneyorothercommodities.Asaresult,thevalueoftheoutputproducedisconsideredtobeabenefit.　　对农户来说，产品的价值与生产要素的成本之差即为利润。就肥料而言，每公顷的利润可表示为　　利润=PY-rX　　其中，P为产品的价格，Y为每公顷产量，r为肥料价格，X为肥料用量。由于产量是肥料用量水平的函数（产量与肥料用量的关系表现在生产函数中），利润与肥料用量水平亦呈函数关系。　　Foranindividualfarmer,thedifferencebetweenthevalueoftheoutputandthecostoftheinputsisreferredtoasprofits.Inthecaseoffertilizer,profitsperhectarecanbewrittenas　　Profits=py-rx　　wherepisthepriceoftheoutput,yistheyieldperhectare,risthepriceoffertilizer,andxistheamountoffertilizerthatisapplied.Sinceyieldisafunctionoftheleveloffertilizeruse(therelationshipbetweenyieldandfertilizeruseisgivenbytheproductionfunction),profitswillbeafunctionoftheleveloffertilizeruse.　　可以把利润看成是收益与成本之差，即：　　利润=收益-成本　　其中，收益=PY，成本=rX。公式中，收益等于所生产的产品的销售收入，成本等于为了生产产品而用于生产要素的费用。注意，固定成本如利息支付或土地支出并不包括在成本里。虽说固定成本很重要，但实际上不能改变，因此在推导取得最大利润的肥料用量时，并不起什么作用。　　Itisusefultothinkofprofitsasthedifferencebetweenbenefitsandcosts,i.e.,　　Profits=Benefits-Costs　　whereBenefits=pyandCosts=rx.Inthisformulation,Benefitsisequaltotherevenueobtainedfromthesaleoftheproductbeingproduced,whileCostsisequaltothemoneythatmustbespentoninputstoproducetheoutput.NotethatfixedcostssuchasinterestpaymentsorlandpaymentsarenotincludedinCosts.Althoughfixedcostsareimportant,thefactthattheyarefixedmeansthattheycannotbealtered.Asaresult,theyplaynoroleinunderstandingtheamountoffertilizertoapplytomaximize\nprofits.　　这里我应该指出，虽然利润是估计农户收益和成本的一个方法，在考虑整个省或整个国家时，需要用其它方法。在这种情况下，收益和成本之差称为纯社会受益。计算纯社会受益时，要考虑到肥料使用的所有受益（包括所创造的就业机会和消费者食物的增加）以及所有成本，例如造成的环境污染。由于这个讲座并不是侧重于纯社会受益，我仅在此强调要用分析利润优化同样的方法来分析这个问题。　　Ishouldpointoutherethatwhileprofitsareawayofvaluingthebenefitsandcostsforanindividualfarmer,othermeasuresneedtobeusedwhenanentireprovinceorcountryisbeingconsidered.Insuchcases,thedifferencebetweenthebenefitsandcostsisknownasnetsocialbenefit.Incalculatingnetsocialbenefit,considerationhastobegiventoallthebenefitsofusingfertilizer(includingthebenefitsfromtheemploymentthatiscreatedandthebenefitsfromconsumershavingadditionalfood),aswellasallofthecostsincludingthecostofthepollutionthatisgenerated.WhileIwillnotfocustoomuchonnetsocialbenefitinthislecture,Iwillstressthatananalysisofthisgoalusesthesamemethodologyasdoesananalysisofprofitmaximization.　　图9表示的是一个假设的水稻生产利润与钾肥用量水平之间的关系。标注“收益”的曲线表示各个钾水平的总收益，由生产函数乘上产品价格而绘出。由于产量对肥料表现为边际报酬递减，收益曲线对肥料也是边际报酬递减。　　Figure9illustratesahypotheticalrelationshipbetweentheprofitsfromgrowingriceandthelevelofpotashfertilizerthatisapplied.ThecurvelabelledBenefitsshowsthetotalbenefitsassociatedwitheachlevelofpotash.Thiscurveisconstructedbyscalingormultiplyingtheproductionfunctionbythepriceoftheoutput.Sinceyieldexperiencesdiminishingmarginalreturnstofertilizer,theBenefitcurvealsoshowsdiminishingmarginalreturnstofertilizer.(图：图9利润与钾肥用量的关系)　　标注“成本”的曲线表示各个钾用量水平的总成本，由肥料用量乘以肥料价格绘制而成。标注“利润”的曲线为收益曲线和成本曲线之差。　　ThecurvelabelledCostsshowsthetotalcostsassociatedwitheachleveloffertilizeruse.Itisconstructedbymultiplyingtheleveloffertilizerusebythepriceoffertilizer.ThecurvelabelledProfitsisthedifferencebetweenBenefitsandCosts.　　因为收益曲线对肥料表现为边际报酬递减，利润曲线也是边际报酬递减。更具体地说，利润曲线的斜率为收益曲线的斜率与成本曲线的斜率之差，这种关系的存在是因为肥料用量的变化量所引起的利润变化，等于由该变化量而产生的收益变化减去成本变化。　　BecausetheBenefitcurveshowsdiminishingmarginalreturnstofertilizer,theProfitscurvealsoshows\ndiminishingmarginalreturns.Morespecifically,theslopeoftheProfitscurveisgivenbythedifferencebetweentheslopeoftheBenefitscurveandtheslopeoftheCostscurve.Thisrelationshipholdsbecausethechangeinprofitsforachangeinfertilizeruseisequaltothechangeinbenefitsforachangeinfertilizeruseminusthechangeincostsforachangeinfertilizeruse.　　收益曲线的斜率称为边际产值（MVP）。边际产值表示肥料用量的变化所引起的收益变化，它等于产品价格乘上边际产量，即：　　MVP=P.MPP　　TheslopeoftheBenefitscurveiscalledtheMarginalValueProduct(MVP).TheMVPshowsthechangeinbenefitsforachangeinfertilizeruse.ItisequaltothepriceoftheoutputtimestheMPP,i.e.,　　MVP=pMPP　　成本曲线的斜率为r，即肥料价格。这是因为，每增加一个单位的肥料用量，成本的增加为r。利润曲线的斜率为MVP-r。　　TheslopeoftheCostscurveisr,thepriceoftheinput.Thisfollowsbecausetheadditionofonemoreunitoffertilizerincreasescostsbyanamountr.TheslopeoftheProfitscurveisthusMVP-r.　　当肥料用量为K*时，利润达到最大。在这一点，利润对肥料用量变化的反应为零，即利润曲线的斜率为零：MVP-r=0。也就是说，在K*这一点，收益曲线的斜率等于成本曲线的斜率，即MVP=P.MPP=r。　　MaximumprofitsoccursatfertilizerlevelK*.Atthispoint,theresponseofprofitstoachangeintheapplicationoffertilizeriszero,i.e.,theslopeoftheProfitscurveiszero?MVP-r=0.ThismeansthatatK*,theslopeoftheBenefitscurveisequaltotheslopeoftheCostscurve-thatis,MVP=pMPP=r.　　这个等式表明，当增加一个单位的肥料用量所得到的收益恰好等于这个单位用量的成本时，利润达到最大。MVP=r可改写为常用的形式　　MPP=r/P　　Thisexpressionsaysthatprofitswillbemaximizedwhenthebenefitfromanadditionalunitoffertilizerisjustequaltothecostofthatunit.ItwillbeusefultorewritetheexpressionMVP=ras　　MPP=r/p　　表1的数据可用来示范怎样运用这一结果。假设水稻价格为0.5元/公斤，钾肥（K2O）的价格为0.73元/公斤，那么钾肥与水稻的价格比便为0.73/0.50=1.46。　　ThedatapresentedinTable1canbeusedtoshowhowthisresultcanbeapplied.Assumethatthepriceofriceis0.5yuan/kg,whilethepriceofpotash(K2O)is0.73yuan/kg.Thismeansthattheratioofthepriceofpotashtothepriceofriceis\n0.73/0.50=1.46.　　如表1所显示，这个比值比早晚稻的边际产量都低，这表明，要取得最大利润，钾肥用量应超过120公斤/公顷。对图2作进一步地分析可得知，钾肥用量在120~180公斤/公顷之间时可能会产生最大利润。这一结论的得出是由于只有在钾肥用量超过120公斤/公顷时，才能使边际产量下降，从而与1.46相等。　　AsTable1indicates,thisratioislessthantheMPPsthathavebeencalculatedforbothlateandearlyrice.Theimplicationisthatpotashapplicationrateswouldhavetobelargerthan120kg/hainordertoobtainmaximumprofitsfromtheuseofpotashfertilizer.Morespecifically,anexaminationofFigure2suggeststhatapotashapplicationlevelofbetween120and180kg/hawouldlikelyresultinmaximumprofits.Thisconclusionisreachedbynotingthatfertilizerapplicationlevelswouldhavetoincreasebeyond120kg/hainordertoreducetheMPPtothepointwhereitwasequalto1.46.　　MPP=r/P的表达式使我们可以比较最大利润与最高产量。如图9所示，取得最大利润的肥料用量(K*)总是低于取得最高产量的肥料用量(K最大)。为了使产量达到最高，边际产量应该为零，而为了获得最大利润，边际产量应该等于肥料和产品的价格比。由于肥料的使用存在着边际报酬递减，增加肥料边际产量的唯一办法是少用肥料，因此，取得最大利润的肥料用量低于取得最高产量的肥料用量。　　TheexpressionMPP=r/pallowsustocompareprofitmaximizationwithoutputoryieldmaximization.AsFigure9illustrates,theleveloffertilizerusethatmaximizesprofits(K*)isalwayslessthanthelevelthatmaximizesoutput(Kmax).Inordertomaximizeyield,theMPPshouldbesetequaltozero.However,tomaximizeprofit,theMPPshouldbesetequaltothefertilizerprice/outputpriceratio.Sincetherearediminishingmarginalreturnstotheapplicationoffertilizer,theonlywaytoincreasetheMPPoffertilizeristouseless.Asaresult,theprofitmaximizingleveloffertilizeruseislessthantheoutputmaximizinglevel.　　MVP=r这个关系是从只使用一种肥料的情况推导出的，但也适用于两种或多种肥料相互作用而影响产量的情况。当使用多种肥料时，需要明确每种肥料的价格和边际产量。例如，如果有两种肥料，肥料价格可分别表示为r1和r2，边际产量可分别表示为MPP1和MPP2，边际价值产品分别为MVP1（等于P?MPP1）和MVP2（等于P.MPP2）。　　Whiletherelationship,MVP=r,wasderivedforthecaseofasinglefertilizer,italsoholdsforthecasewheretwoormorefertilizersinteracttoaffectyield.Whenthereismorethanonefertilizer,itisnecessarytospecifythepricesandthemarginalphysicalproductsforeachofthefertilizers.Forinstance,iftherearetwofertilizers,thepricesofthesefertilizerscouldbespecifiedasr1andr2,whilethemarginalphysicalproductscouldbespecifiedasMPP1andMPP2.ThemarginalvalueproductsareMVP1(equalspMPP1)andMVP2(equalspMPP2).　　为了获得最大利润，两种肥料的用量都必须是在MVP1=r1和MVP2=r2的时候，换言之，　　MPP1=r1/P,MPP2=r2/P　　由于一种肥料的用量水平会影响到另一种肥料的边际产量，需要调节两种肥料的用量水平直到满足以上两个等式。　　Tomaximizeprofits,itisnecessarytofindthelevelofbothfertilizerswhereMVP1=r1andMVP2=r2,oralternatively,where　　MPP1=r1/pandMPP2=r2/p　　SincethelevelofonefertilizeraffectstheMPPoftheother,itisnecessarytoalterthelevelsofbothfertilizersuntiltheaboveequalityis\nobtained.　　图10中，获得最大利润的氮和钾用量分别为N*和K*。这个用量是由MPPN=rN/P和MPPK=rK/P而得出的。请注意到N*和K*都低于最高产量的用量水平N最大和K最大。　　Figure10showsthattheprofitmaximizinglevelsofnitrogenandpotashareN*andK*.TheselevelsarefoundbeequatingrN/pandrK/pwithMPPNandMPPK,respectively.NotethatbothN*andK*arelessthanNmaxandKmax,thelevelsofnitrogenandpotashthatmaximizeyield.(图：图10两种肥料的生产函数达到最大利润的肥料用量水平)　　以上结果表明，只有在肥料价格为零时，最大利润才会和最高产量相一致，换言之，只有在肥料价格为零时，最高产量才有经济学上的意义。　　Theimplicationoftheaboveresultisthatunlessthepriceoffertilizeriszero,themaximizationofoutputisnotconsistentwithmaximizingprofits.Or,putsomewhatdifferently,maximizingoutputonlymakessensefromaneconomicperspectivewhenthepriceoffertilizeriszero.　　经验证据EmpiricalEvidence　　产量优化与利润优化的问题很重要，因为我所参与的一项研究表明，至少在中国的某些地区，农户仍倾向于把氮肥用到最高产量的水平。由于肥料价格通常并不为零，最高产量意味着氮肥的使用已过量。这表明，可用于其它生产投入如钾或用于购买农产品或家用产品的资金被用在了氮肥上，其结果是农户所获得的利润减少。　　ThequestionofoutputversusprofitmaximizationisimportantsinceresearchthatIhavebeeninvolvedwithshowsthatfarmersinatleastsomepartsofChinahavetendedtousenitrogenfertilizertothepointwhereoutputismaximized.Sincethepriceoffertilizerisusuallynotzero,themaximizationofoutputmeansthattoomuchnitrogenisbeingused.Theuseoftoomuchnitrogenmeansthatresources(e.g.,money)arebeingspentonnitrogenthatcouldbespentonotherinputssuchaspotash,orusedinthepurchaseofotherfarmorhouseholdproducts.Theresultisareductioninthelevelofprofitsthatcouldbeearnedbyfarmers.　　举例而言，表3是1988年湖南省中东部地区54个农户的施肥情况。其中，10户位于高产地区，早稻平均亩产达1045斤/亩。10户中9户用氮肥，5户用磷肥，6户用钾肥，9户用粪肥。　　TogiveanexampleoffertilizeruseinChina,Table3presentsdatacollectedfrom54farmsineast-centralHunanprovinceregardingtheiroperationsin1988.Tenofthefarmsarelocatedinahighyieldregion,where\ntheaverageyieldofearlyriceis1,045jin/mu.Ofthe10farmsinthisarea,9appliednitrogen,5appliedphosphate,6appliedpotash,and9usedmanure.(表：表3早稻的产量与肥料的用量（1988年，湖南省中东部地区）)Range产量幅度Average平均值#Fertilizer施肥户数MPP边际产量Stat.Diff.FromZero与0的统计学差异(jin/mu)HighYieldRegion(10observations)高产区（10个观察值）Yield产量900-12001045.0---Nitrogen氮0~5735.69-0.19No无Phosphate磷0~5022.550.22No无Potash钾0~208.760.82No无Manure有机肥0~40002000.090.004No无LowYieldRegion(44observations)低产区（44个观察值）Yield产量500~1000709.1---Nitrogen氮15~6235.7442.44No无Phosphate磷0~10043.1320.01No无Potash钾0~306.2191.26YES有Manure有机肥0-100002657.0400.001No无注释：N是尿素，P是过磷酸钙，K是氯化钾。　　在低产区的早稻亩产为500~1000斤/亩，虽然44户均施用氮，只有19户施钾，32户施磷。平均用氮水平为35.7斤/亩，与高产地区相接近。平均磷用量为43.1斤/亩，明显地高于高产地区的22.5斤/亩。然而，在施用磷肥的农户中，差别却小得多：高产区为45.0斤/亩，低产区为59.3斤/亩。　　Inthelowyieldregion,earlyriceyieldsrangedfrom500to1,000jin/mu.Whileall44farmersintheregionappliednitrogen,only19appliedpotash.Thirty-twofarmersappliedphosphate.Theaveragelevelofnitrogenappliedwas35.7jin/mu,aboutthesameasinthehighyieldregion.Theaveragelevelofphosphateapplicationwas43.1jin/mu,considerablyhigherthanthe22.5jin/mulevelinthehighyieldregion.However,amongthosefarmersthatusedphosphate,thedifferencewasmuchless:45.0jin/muinthehighyieldregionascomparedto59.3jin/muinthelowyieldregion.　　在高产区，施用钾肥的农户平均用量为14.5斤/亩，与低产区的14.4斤/亩接近。　　Inthehighyieldregion,theaveragepotashuseamongfarmersusingpotashwas14.5jin/mu,almostthesameasthe14.4jin/muaverageamongthefarmersthatusedpotashinthelowyieldregion.　　表3还显示了以上两个地区四种肥料的边际产量。在高产区，四种肥料的边际产量都很小，统计上基本上是等于零，这说明，该地区的肥料用量与获得最大利润时所需要的用量相比已过量。　　Table3alsopresentstheMPPsthatwereestimatedforthefourdifferentfertilizersineachofthetworegions.Inthehighyieldregion,theMPPsofallthefertilizersaresmallandcannotbedifferentiatedfromzerostatistically.Theimplicationisthatfertilizerisgenerallybeingoverusedinthisregioncomparedto\nthelevelthatwouldmaximizeprofits.　　在低产地区，磷肥和粪肥的边际产量都很小，统计上接近于零，说明这两种肥料的用量接近于最高产量的用量水平。由于粪肥的价格可以看成是很低，这种养分的使用达到边际产量为零的水平并不奇怪；然而，磷肥的价格并不为零，磷肥的使用显然已经过量。　　Inthelowyieldregion,theMPPsofphosphateandmanurearesmallandcannotbedifferentiatedfromzerostatistically.Thisimpliesthatthesetwofertilizersarebeingusedatalevelthatwouldmaximizeyield.Sincethepriceofmanurecanbeconsideredtobeverylow,itisnotsurprisingthatthisnutrientsourceisbeingusedtothepointwheretheMPPequalszero.However,giventhatthepriceofphosphateisnotzero,itwouldappearthatthisfertilizerisbeingoverused.　　对氮肥边际产量的测算相对较高，但由于数据的变异性，这个测算并不十分准确。因此可以排除氮肥的边际产量接近于零的可能，但不能排除其等于氮肥与水稻的价格比的可能。　　TheestimateoftheMPPofnitrogenisrelativelylarge.However,becauseofvariabilityinthedata,thisestimateisnotveryprecise.Asaresult,thepossibilitythattheMPPiszerocannotberejected.Neither,however,canthepossibilitythattheMPPisequaltothenitrogen/ricepriceratioberejected.　　对钾肥边际产量的估计是正值，而且统计上显然不等于零。这表明在追求最高产量时钾肥的使用并未过量。由于大部分农户并不用钾肥，为了了解农户用钾是否符合最大利润用量，对此进行了统计检验。检验结果表明确实如此。因此，对于那些未使用钾肥的农户，使用钾肥会增加他们的利润，也就是说，生产函数估计表明，不用钾肥不能取得最大利润。　　TheestimateoftheMPPofpotashispositiveandstatisticallydifferentfromzero.Thisimpliesthatpotashwasgenerallynotbeingoverusedinanattempttomaximizeproduction.Sincealargenumberoffarmersdidnotusepotash,statisticaltestswerecarriedouttoseeifthefarmersthatusedpotashdidsoinamannerthatwasconsistentwithprofitmaximization.Itwasfoundthatthiswasthecase.Forthefarmersthatdidnotusepotash,however,accesstopotashfertilizerwouldhaveincreasedtheirprofits,ie.,theproductionfunctionestimatesindicatethatzeropotashusedoesnotmaximizeprofits.　　然而，如大家所知，农户能购买和使用的肥料量常常受到限制。在存在限制因素的情况下，合理施肥的经济问题也发生了变化。下一讲座的题目就是在存在限制条件的情况下怎样进行分析。　　Unfortunately,asyouknow,farmersareoftenconstrainedintheamountoffertilizerthattheycanpurchaseoruse.Whenconstraintsareinplace,theeconomicsoffertilizationchanges.Thesubjectofmynextlecturewillbehowtoanalyzesituationsinwhichconstraintsarepresent.