planar kinematics of a rigid body review刚体平面运动的回顾 16页

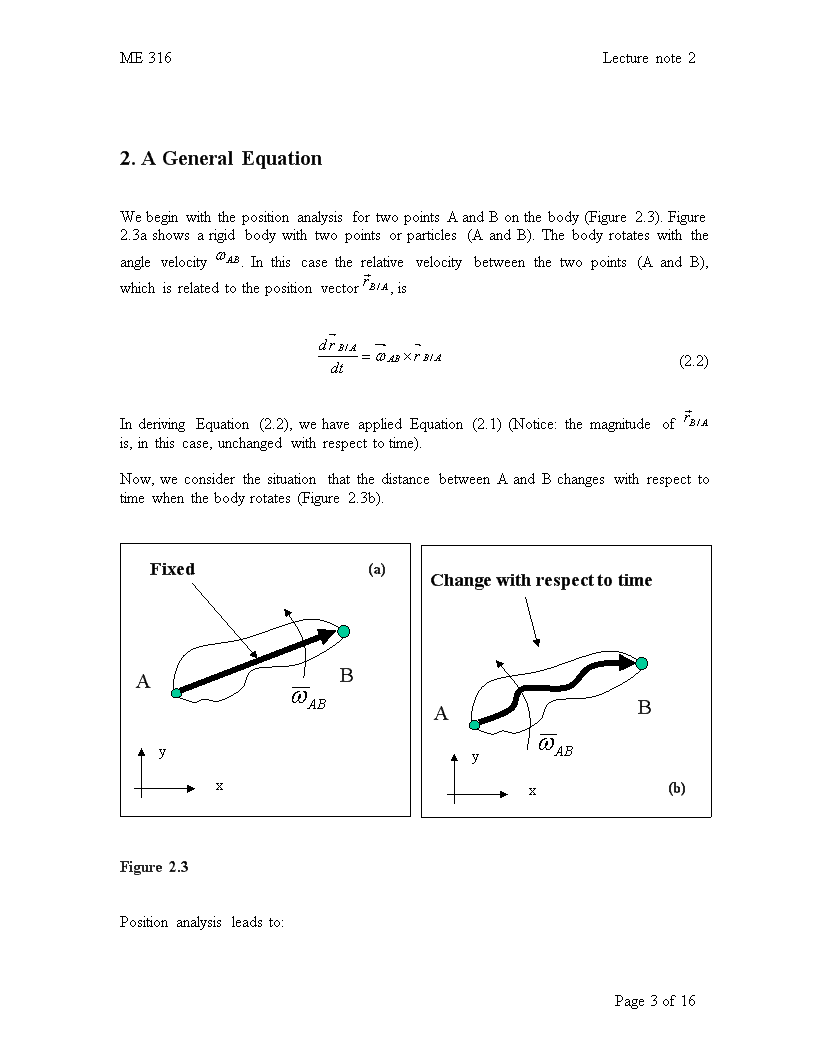
ME316Lecturenote2LectureNote(2)AGeneralMethodforKinematicsofPlanarMechanismsTopic:1.DerivativeofaVectorwhenitrotates2.Ageneralequation3.ExamplesRemarks:Alsorefertothetext(9thEdition)(pp354-369).1.DerivativeofaVectorwhenitrotatesLetbeavector.Itsmagnitudeisconstant.Vectorrotatesaboutanaxisperpendiculartothesheetplanewithanangularvelocity;seeFigure2.1a.Notethatintheplanarmotionthedirectionoftheangularvelocitywillbeperpendiculartothesheetplane;seeFigure2.1b.(b)(a)xyzFigure2.1Page16of16\nME316Lecturenote2Wecanprovethefollowingequation:(2.1)Proof:ThederivativeofthevectorAwithrespecttotimecanbewrittenasfollows:wherethemagnitudeofcanbeexpressedas()A;seeFigure2.2.Thedirectionofthelimitcanbeexpressedbywherethefirstunitvectoristherotationaxisperpendiculartothesheetplaneandoutward,whilethesecondunitvectoristhedirectionof.ThereforewecanobtainFigure2.2Endoftheproof.Notethattheaboveproofcanbefoundfromthetext(P355,9thEdition).Page16of16\nME316Lecturenote22.AGeneralEquationWebeginwiththepositionanalysisfortwopointsAandBonthebody(Figure2.3).Figure2.3ashowsarigidbodywithtwopointsorparticles(AandB).Thebodyrotateswiththeanglevelocity.Inthiscasetherelativevelocitybetweenthetwopoints(AandB),whichisrelatedtothepositionvector,is(2.2)InderivingEquation(2.2),wehaveappliedEquation(2.1)(Notice:themagnitudeofis,inthiscase,unchangedwithrespecttotime).Now,weconsiderthesituationthatthedistancebetweenAandBchangeswithrespecttotimewhenthebodyrotates(Figure2.3b).ABFixedChangewithrespecttotime(a)AB(b)xyxyFigure2.3Positionanalysisleadsto:Page16of16\nME316Lecturenote2(2.3)ThefirstorderderivativeofEquation(2.3)onbothsideswithrespecttimeleadsto:(2.4)TheseconditemontherightsideofEquation(2.4)isfurtherwritteninto:(2.5)whereisanunitvectorrepresentingthedirectionofvector.ThephysicalmeaningoftheitemsontherightsideofEquation(2.5)canbeexplained.Thefirstitem:therateofchangeinthedistancebetweenAandB,i.e.,therelativetranslationvelocitybetweentwopoints(A,B).Theseconditem:therateofchangeofaunitvectorwhoseorientationchangesbutnotitslengthormagnitude.AccordingtoEquation(2.1),weobtain:(2.6)BringingtogetherEquations(2.4)-(2.6),weobtain(2.7)(2.8)where:theabsolutevelocityofpointB:theabsolutevelocityofpointAPage16of16\nME316Lecturenote2or:therelativetranslationvelocitybetweenpointsAandB,alsocalled“stretch”term:therelativerotationvelocitybetweenpointsAandB,alsocalled“rotational”termTheaccelerationequationcanbederivedbydifferentiatingEquation(2.7).Inthedifferentiationprocess,weneedtonoticethatthefollowingvectorschangetheirorientationonly:;thefollowingvectorschangeboththeirorientationandmagnitude:,;thefollowingscalarquantitychangeswithrespecttotime:.(2.10)where:thestretchorrelativetranslationacceleration.:theCoriolisacceleration.:theCentripetalacceleration:theaccelerationduetothechangeinmagnitudeoftheangularvelocity.TypicalmechanicalconstructionpatternsforthesituationshowninFigure2.3bareillustratedinFigure2.4.Wenotethatinbothcases(Figure2.4),points(A,B)areondifferentbodies(1,2),respectively.Page16of16\nME316Lecturenote2BAABABAB2112Figure2.4ExampleExample1(Figure2.5).ThereisapivotatpointAbetweenbarABandtheflatroad.Voisconstant,andθisknown.Find:theangularvelocityofBarAB,ω(θ),andα(θ).ABV0θ12aFigure2.5Solution:Page16of16\nME316Lecturenote2Analysis:Theknownmotionvariableisonbody2(i.e.,V0),andtheunknownvariableisonbody1.Sothereisaneedtoestablishaconnectionbetweenbody1andbody2.ThecontactpointBwillbeusedforthispurpose.OurgeneralideaistoapplyEquation(2.8)tobuildsuchaconnection.Forthispurposeweneedtochooseproperlytwopoints.HerewechoseonepointB(onbody2)andonepointA(onbody1).Itisobviousthatthechosenpoints(A,B)fitthepatterntowhichEquation(2.8)canbeapplied(seealsoFigure2.4b).Thedetailedsolutionisgivenbelow.Step1:ChooseB(onbody2)andA(onbody1)Step2:BecausethedistancebetweenBandAchangeswithrespecttotime,weapplyEquation(2.8),particularly,Intheaboveequation,wecanestablishananalysistable.=++´Dir.√√√√√Mag.√√??√Intheabovetable,√:means“known”?:means“unknown”Fromtheabovetable,weknowthatwehavetwounknowns.Wehavetwoequations.Therefore,weareconfidentthattheproblemcanbesolved.Step3:VectorEquation(a)canbewrittenintotwoscalarequations,i.e.,Page16of16\nME316Lecturenote2From(c),Substituting(d)into(b)givesBut(f)So(g)Alsobysubstitutingequation(g)intoequation(c),weobtain,Page16of16\nME316Lecturenote2AccelerationAnalysis(j)Multiplyingequation(i)bysinqandequation(j)bycos(q)gives,respectively,andsubtracting(l)from(k)gives(m)But,fromequations(f),(g)&(h)Page16of16\nME316Lecturenote2(n)(o)(p)Sosubstituting(n),(o),(p)intoequation(m)givesExample2(Figure2.6)Figure2.6showstheschematicdiagramofamechanism.Thedimensionsofthemechanismareindicatedonthefigure.isknown.FindCABE1’’2’’D0.5’’2rad/syxFigure2.6Page16of16\nME316Lecturenote2Solution:Analysis:TheknownmotionvariableisonBarBD,whiletheunknownmotionvariableisonBarCE.PointsAandBarecoincidentatthelocationofcontactbetweentwobars.Thereforethereisaneedtoestablishaconnectionequationbetweentwobars(i.e.BD&CE).Again,thekeystepnowistochoosetwopointsfromthesystemsuchthatoneappearsonBarCEandtheotheronBarBD.Thebestchoicehereis:ConBarCEandBonBarBD.Thedetailedsolutionisgivenbelow.Step1:BychoosingCandBarCEandBonBarBD,wecanobtain:(a)Weestablishatabletoseeifwecanfindthesolution.Dir.√√√√√√Mag.√√?√?√Fromtheabovetable,wecanseethenumberofunknownsis2,andwehavetwoscalarequations,sowecansolvethisproblem.Notethatisknownbecauseisgiven.Thedetailedsolutionisgivenbelow,Step2:PickBandDonbody2,wehave(b)Thatis,(c)Page16of16\nME316Lecturenote2Step3:PickupConBody1andBonBody2.BecausethedistancebetweenCandBchangeswithrespecttotime,weapplyEquation(2.8).Thatistosee,wegetequation(a);seethepreviousdiscussion.Writeequation(c)intothescalarequations,or(d)(e)orPage16of16\nME316Lecturenote2SomeremarksregardingExample2arefurthergivenasfollows:Remark1.Why?Explanation:Wefirstreviewthedefinitionoftheangularvelocityofanobjectthatisrepresentedbytwopoints(seeFigure2.7).InFigure2.7weshowtwopoints:PandM.Theleftdiagramshowstheirpositionatoneinstant,sayt1.Atanotherinstant,sayt2,bothpointsmovetoanewposition,P’andM’,respectively.TheangularvelocityisdefinedastherateofchangeintheorientationoflinePM;thatis,}{lim0ttPMDD=®DqwLetusreturntoExample2.Figure2.8showsthefollowing:(1)AtaninstancethatrodBDandrodCEareincontact.TheircontactpointsareA1andB,respectively.A1isapointonrodCE(orBody1).(2)AtanotherinstancethatrodBDandrodCEareincontact.TheirnewcontactpointsareA1andB’.A1isapointonrodCE.B’isapointonrodBD.Nowletusexamine:and,respectively.:Twopointswhichareinvolvedintheangularvelocityare:BandD.TheangularvelocitywhichistheangularvelocityofrodBD.:Twopointswhichareinvolvedinangularvelocityare:A1andB.Itisnotedthatattwoinstances,thepointonrodCE,whichisincontactwithrodBD,isA1andA1’,respectively.Accordingtoourpreviousdiscussionontheangularvelocityingeneral(seeFigure2.7),weknowthattheangularvelocityof“rod”AD(thereisnotanyphysicalrodexistedbetweenAandDandtherodADisanimaginaryonethere)shouldbetherateofthechangeintheanglebetweenlineDA1andDA1’.Page16of16\nME316Lecturenote2isrelatedtothechangeinangle∆α,whileisrelatedtothechangeinangle∆θ.Becausethesetwochangesinanglearedifferent,.Figure2.7∆αA2(B’)A1(B)A1’EDCθ∆θFigure2.8Page16of16\nME316Lecturenote221Figure2.9Remark2.Why?Explanation:Attimeinstant,sayt1,theconfigurationofthemechanismisshowninFigure2.9a.ThecontactpointfromrodCEisA1.Attimeinstant,sayt2,A1movestoA1’,andEmovestoE’(Fig.9b).Notethatthecontactbetweenbody1andbody2hasbeenchangedduringthemotion.InFig.9b,thecontactisA2notA1.Inanycase,itisclearthatwewillalwayshave:.Thismeans.Remark3.Why?Weexaminethesituationattwotimepoints;seeFigure2.10.Fromthisfigurewecanobtain:Page16of16\nME316Lecturenote2BB’CEE’Figure2.10Therefore,theangularvelocityoflineCBisthesameastheangularvelocityofbarCE.Page16of16